KOMPSAT-5 L1 calibration

Ingested KOMPSAT-5 L1D products are systematically calibrated in the ESA Charter Mapper using the Radar Products Calibration (SAR-Calib) processor.

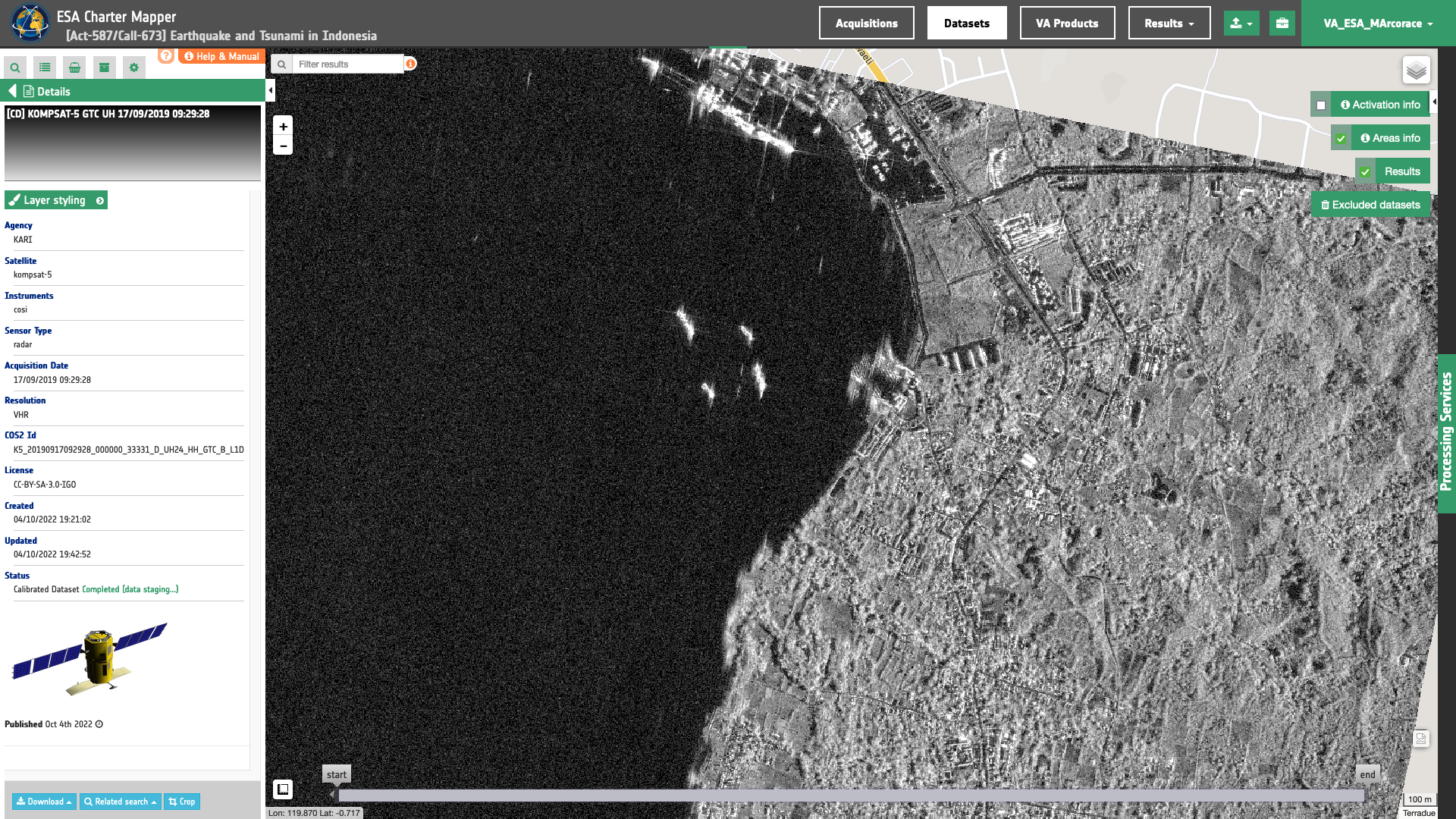
KOMPSAT-5 sigma nought

Figure 1 - Full resolution grayscale overview from a KOMPSAT-5 GTC UH product in HH polarization acquired in 2019 near Pantoloan in Tawaeli, Central Sulawesi, Indonesia. Image credit: KARI.

Input

The input is a KOMPSAT-5 GTC Dataset derived from an ingested KOMPSAT-5 Level-1D product, see details here.

Workflow

The workflow for the systematic generation of Radar Calibrated Datasets from KOMPSAT-5 GTC L1D products is described in the below graph.

graph TB subgraph Input style Input fill:#e8e8e8,stroke:#333,color:#282828 a1[/KOMPSAT-5 L1D Dataset/] style a1 fill:#acc8ff,stroke:#333,color:#282828,stroke-width:2px end subgraph SAR-Calib a1 --> calib[Radiometric Calibration] calib --> ml[Multilooking] style ml fill:#cfdfff,stroke:#333,color:#282828 ml --> ltodb1[Linear to dB] ltodb1 --> cog1[Convert to COG] cog1 --> p1(Sigma Nought) style p1 fill:#cfdfff,stroke:#333,color:#282828 ml --> despeckle[Speckle Filtering] despeckle --> ltodb2[Linear to dB] ltodb2 --> overcreat[Image stretching and RGB composite creation] overcreat --> cog2[Convert to COG] cog2 --> p2(overview/s) style p2 fill:#cfdfff,stroke:#333,color:#282828 p1 --> stac[Create STAC item] p2 --> stac[Create STAC item] end subgraph Output style Output fill:#e8e8e8,stroke:#333,color:#282828 stac --> out[/KOMPSAT-5 L1D Calibrated Dataset/] style out fill:#acc8ff,stroke:#333,color:#282828,stroke-width:2px out --> o1[/s0_db_x_hh, or s0_db_x_hv, s0_db_x_vh, s0_db_x_vv/] style o1 fill:#acc8ff,stroke:#87afff,color:#282828,stroke-width:2px out --> o2[/overview-hh, or overview-hv, overview-vh, overview-vv/] style o2 fill:#acc8ff,stroke:#87afff,color:#282828,stroke-width:2px end

This diagram represents the application of the SAR-Calib processor workflow applied for KOMPSAT-5 L1D Datasets. More details about this systematic processor can be found here.

Warning

The calibration of KOMPSAT-5 L1D GTC products in Wide Swath or Enhanced Wide Swath mode is not currently supported.

Radiometric calibration

The Radiometric Calibration step of the workflow applies the sigma nought equation for KOMPSAT-5 L1D products¹. Sigma nought in logarithmic scale (dB) can be derived from KOMPSAT-5 GTC L1D products using the following expression:

\[ \sigma_0(dB) = 10 \times log_{10}(K \times DN^2) \]

where \(DN\) is the Digital Number and \(K\) is the calibration factor which is derived with the following equation:

\[ K = { CALCO \over cellsize } \times | RF^2 | \]

in which:

\(RF\) is the Rescaling Factor, derived from product metadata file under RescalingFactor,
\(CALCO\) is the Calibration Constant, included in the metadata at CalibrationConstant,
and \(cellsize\) is obtained by multiplying the values of ColumnSpacing and LineSpacing available in the product annotation.

Multilooking

Details about the Multilooking step can be found here.

Overview creation

For Image stretching and RGB composite creation step refer to sections about signal dynamic ranges for stretching sigma nought and overview creation sections.

Output

The output is a KOMPSAT-5 GTC Calibrated Dataset represented by a STAC item GeoJSON² including:

single-band assets for backscatter at each polarization,
and multi-band assets for visual products (single-pol overviews and dual- or full-pol band composites).

All assets are given at full resolution and in COG format.

Backscatter products are Float32 single-band assets in COG format, one for each polarization (e.g. s0_db_x_vv which refers to the common band name for sigma nought in dB from X-band SAR in VV polarization).

Visual products are given as 8-bit multi-band assets in COG format (e.g. overview-vv which refers to speckle filtered visual product at full resolution derived from sigma nought in dB from X-band SAR in VV polarization). A default overview product at low resolution is also derived for product preview when EULA is not signed by the user.

As an example, below is reported an extraction of the STAC item for a Calibrated Dataset from KOMPSAT-5 L1D GTC SM in VV polarization acquired in the Charter Activation 787.

    "assets": {
    "s0_db_x_vv": {
      "type": "image/tiff; application=geotiff",
      "roles": [
        "data",
        "sigma0",
        "visual"
      ],
      "href": "https://.../K5_20221009231907_000010_50150_A_ST08_VV_GTC_B_L1D_Aux_xml-calibrated/s0_db_x_vv.tif",
      "sar:polarizations": [
        "VV"
      ],
      "raster:bands": [
        {
          "spatial_resolution": 3.3333333333333335,
          "statistics": {
            "minimum": "-48.060546875",
            "maximum": "0.15378950536251",
            "mean": "-9.7672014822465",
            "stddev": "7.1935299999715",
            "valid_percent": "70.45"
          },
          "histogram": {
            "count": 256,
            "min": -48.155086517333984,
            "max": 0.24832741916179657
        }
      "file:size": 396903957
    },
    "overview-vv": {
      "type": "image/tiff; application=geotiff",
      "roles": [
        "composite",
        "sigma0",
        "visual"
      ],
      "href": "https://.../K5_20221009231907_000010_50150_A_ST08_VV_GTC_B_L1D_Aux_xml-calibrated/overview-vv.tif",
      "sar:polarizations": [
        "VV"
      ],
      "raster:bands": [
        {
          "spatial_resolution": 3.333333333333333,
          "statistics": {
            "minimum": "1",
            "maximum": "234",
            "mean": "146.52680880641",
            "stddev": "51.624258414892",
            "valid_percent": "70.45"
          },
          "histogram": {
            "count": 256,
            "min": 0.5431372549019609,
            "max": 234.45686274509805
          }
        },
        {
          "spatial_resolution": 3.333333333333333,
          "statistics": {
            "minimum": "255",
            "maximum": "255",
            "mean": "255",
            "stddev": "0",
            "valid_percent": "70.45"
          },
          "histogram": {
            "count": 256,
            "min": 254.5,
            "max": 255.5
          }
        }
      ],
      "file:size": 95616809
    },
    "overview-vv-low-res": {
      "type": "image/tiff; application=geotiff",
      "roles": [
        "composite",
        "overview"
      ],
      "href": "https://.../K5_20221009231907_000010_50150_A_ST08_VV_GTC_B_L1D_Aux_xml-calibrated/overview-vv-low-res.tif",
      "s_expressions": "",
      "raster:bands": [
        {
          "spatial_resolution": 16.666666666666664,
          "statistics": {
            "minimum": "0",
            "maximum": "233",
            "mean": "103.22112050398",
            "stddev": "79.679079286019",
            "valid_percent": "100"
          },
          "histogram": {
            "count": 256,
            "min": -0.4568627450980392,
            "max": 233.45686274509805
          }
        },
        {
          "spatial_resolution": 16.666666666666664,
          "statistics": {
            "minimum": "0",
            "maximum": "255",
            "mean": "179.60778473312",
            "stddev": "116.36592615627",
            "valid_percent": "100"
          },
          "histogram": {
            "count": 256,
            "min": -0.5,
            "max": 255.5,
          }
        }
      ],
      "file:size": 9531342
    }
  }
}

SI Imaging Services, Kompsat-5 Sigma Naught Equation, available at: www.si-imaging.com. ↩
Radiant Earth Foundation, STAC item specifications, a single spatiotemporal asset as a GeoJSON feature plus datetime and links, available at:https://github.com. ↩